The Bayes Theorem Significance
28 May 2018Prologue To The Bayes Theorem Significance
The Bayes Theorem Significance
$\;\;\;\;P(B_{i}\vert A)$=$\frac {P(A\vert B_{i})\cdot P(B_{i})}{P(A\vert B_{1})\cdot P(B_{1})+…+P(A\vert B_{n})\cdot P(B_{n})}$
[1] The general form of Bayes theorem embedes a total probability expression in its denominator part.➀such total probability is the linear combination of the events in the sample space with the probability $P(A\vert B_{i})$, the occurrence of the target condition $A$, under the given partitioned sample space $B_{i}$ as it’s weighting.
[2] The general form of Bayes theorem has joint probability expression in its nominator part.
➁usually, $A$ is the qualitative feature of interest.
➂$\Omega$=$\{B_{1},B_{2},…,B_{n}\}$, where $B_{i}$ is the partition os the sample space.➀the joint probability is expressed in terms of $P(A\vert B_{i})\cdot P(B_{i})$.
[3] Take the Bayes theorem into parts
➁$P(A\vert B_{i})\cdot P(B_{i})$=$P(A\cap B_{i})$, is to calculate the possible likelihood of the probability of the coexistence of $A$ and $B_{i}$.There exists 4 factors in the Bayes theorem expression.
➀$B_{i}$ is the prior probability, the already known probability.
➁$P(A\vert B_{i})$ is the likelihood function, by intuition the qualitative term, its major effect is the estimation of the possible likely probability of the occurrence of target event of interest $A$, under the given condition/partition $B_{i}$ of sample space.
➂the total probability also called the marginal probability, it is the summation over the distribution of each distinct $B_{i}$ with regards to the specific $A$, that means there could be infinite numbers of $A$.
➃$P(B_{i}\vert A)$ is the posterior probability, by intuition the quantitative target, now the sample space is the target event of interest $A$.
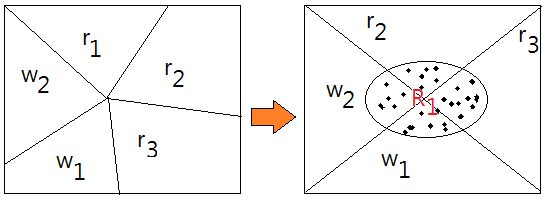
Example: 3 Red And 2 White Balls
[Question]Given that there are 3 red and 2 white balls in a bow. Suppose you pick up 2 balls sequentially. What’s the probability of picking up the 2nd white ball and the 1st one is the red ball?
[Answer]
This is asking for $P(W_{2}\cap R_{1})$. Denote $R_{i}$,$W_{i}$ as the i-th picking the red, white ball up.
[More details]
➀when we pick up the very first ball, $\Omega$=$\{r_{1},r_{2},r_{3},w_{1},w_{2}\}$.
$P(R_{1})$=$\frac {3}{5}$, the probability that the 1st ball is a red ball.
➁when we pick up the second ball, $\Omega$=$\{r_{2},r_{3},w_{1},w_{2}\}$.
$P(W_{2}\vert R_{1})$=$\frac {2}{4}$, the probability that the 2nd ball is a white ball, given that the first one is the red ball.
➂$P(W_{2}\cap R_{1})$=$P(W_{2}\vert R_{1})\cdot P(R_{1})$=$\frac {6}{20}$.➀$P(R_{1})$ is the prior priority.
➁$P(W_{2}\vert R_{1})$ is the likelihood function to estimate the probability that the 2nd ball is a white ball, given that the first one is the red ball.
➂$P(W_{2}\vert R_{1})\cdot P(R_{1})$ is the joint probability of the coexistence of $W_{2}$ and $R_{1}$, since we are asking for the probability of picking up the 2nd white ball and the 1st one is the red ball.
Example: quantitative versus qualitative::mjtsai1974
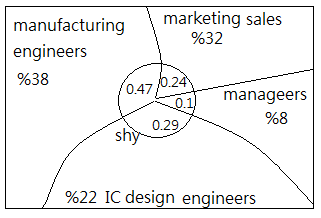
[Question]Suppose the statistical population distribution in the Hsin-Chu science park area reports that $\%8$ of the population is the managers, $\%32$ is the marketing sales, $\%38$ is the manufacturing engineers, $\%22$ is the IC design engineers.
Given that Albert is a man, when you see him, his behavior is a little shy, talktive with strangers for road seeking, and is not talktive in political topics.
According to recent statistical research that given a manager, $0.1$ probability being shy; given a marketing sales, $0.24$ probability being shy; given a manufacturing engineer, $0.47$ probability being shy; given an IC design engineer, %0.29% probability being shy.
Under such condition, given that Albert is a shy man, what kind of identity has the maximum probability?[Answer]
➀take $P(Mgr)$=$0.08$, $P(Sales)$=$0.32$, $P(M_{eng})$=$0.38$, $P(I_{eng})$=$0.22$ for probability of being managers, marketing sales, manufacture engineers, IC design engineers, which are all the prior probability.
➁take $P(Shy\vert Mgr)$=$0.1$, $P(Shy\vert Sales)$=$0.24$, $P(Shy\vert M_{eng})$=$0.47$, $P(Shy\vert I_{eng})$=$0.29$ for given a manager, a marketing sales, a manufacturing engineer, IC design engineer, the probability being shy respectively, which are all the likelihood function, the qualitative term.
➂the question asks for the maximum in between $P(Mgr\vert Shy)$, $P(Sales\vert Shy)$, $P(M_{eng}\vert Shy)$, $P(I_{eng}\vert Shy)$. Trivially, we need the total probability of being shy.
$P(Shy)$
=$P(Shy\vert Mgr)\cdot P(Mgr)$+
$\;\;\;\;P(Shy\vert Sales)\cdot P(Sales)$+
$\;\;\;\;P(Shy\vert M_{eng})\cdot P(M_{eng})$+
$\;\;\;\;P(Shy\vert I_{eng})\cdot P(I_{eng})$
=$0.1\cdot 0.08$+$0.24\cdot 0.32$+$0.47\cdot 0.38$+$0.29\cdot 0.22$
=$0.3272$
➃finally, the posterior probability:
$P(Mgr\vert Shy)$=$\frac {P(Shy\vert Mgr)\cdot P(Mgr)}{P(Shy)}$=$\frac {0.1\cdot 0.08}{0.3272}$=$0.0244$
$P(Sales\vert Shy)$=$\frac {P(Shy\vert Sales)\cdot P(Sales)}{P(Shy)}$=$\frac {0.24\cdot 0.32}{0.3272}$=$0.2347$
$P(M_{eng}\vert Shy)$=$\frac {P(Shy\vert M_{eng})\cdot P(M_{eng})}{P(Shy)}$=$\frac {0.47\cdot 0.38}{0.3272}$=$0.5458$
$P(I_{eng}\vert Shy)$=$\frac {P(Shy\vert I_{eng})\cdot P(I_{eng})}{P(Shy)}$=$\frac {0.29\cdot 0.22}{0.3272}$=$0.1949$By means of quantitative posterior, we found that $P(M_{eng}\vert Shy)$ has the largest posterior probability and implies the maximum possibility that Albert is a shy man, he is a manufacture engineer.